The question how much advantage the right of the first move implies has always been of interest for philosophical minds. But there is also a lot of practical importance in finding the right answer.
For instance, some people might want to know, how much it hurts them if they have to play with Black in over 50% of the games in team competitions when players in front of them pause in an unfavourable way.
Or, what exact difference in Elo points between both players would make a draw an Elo-neutral result? The answer requires the exact information about the expected value of the respective colours. I never bothered to do a calculation here, but my vague guess would be that if the white player is weaker by 100 points, this roughly compensates his first mover advantage. Consequently, a draw with Black against someone with 150 points less is still not the end of the world. However, when the difference is 200 points you might want to think about playing the right line with Black in order to avoid a draw.
Most importantly, we have to know the proper benchmark in order to recognize whether a specific variation is an out- or underperformer in a statistical sense. I will deal with that important topic in future articles.
Today we are in the enviable situation of being capable of making assumptions about the equity distribution of both colours, which are supposed to be extremely close to reality. Let’s have a look at the following table, which is taken from the CHESSBASE Online Database. Note that the percentage values are always from White’s perspective. The lower the figures, the better for Black.
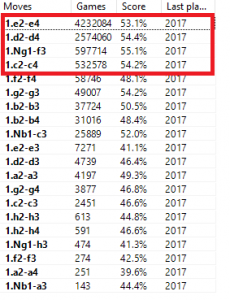
If you consider the four most relevant moves, you see that the approximate average is a score of 54% by White.
Whenever you deal with statistics be aware of potential distortions. By looking at the plain numbers one could assume that 1.Nf3 is the best move and 1.e4 is worse than the rest. I don’t believe that this is the case. I rather think all of these moves have more or less the same value. With regard to 1.e4, let’s not forget that this is the typical beginner’s move. Therefore, the quality of the players here is below average. Hence, I call this the beginner’s bias.
As for 1.Nf3, there very likely is the opposite effect in place, namely the expert’s bias. Most beginner’s either play 1.e4 or 1.d4, so the level of the 1.Nf3 protagonists is higher than average because of this fact alone. But there is more. Many 1.Nf3 players follow up with 2.d4, 2.c4 or 2.g3, depending on Black’s answer. That way, they circumvent many lines which could occur after a direct 1.d4, 1.c4 or 1.g3, and which they may consider unpleasant. Alternatively, they might just want to prevent Black from getting what he wants or where he is an expert in. If you play 1.Nf3 and 2.d4 (or the other way round) and c4 only in the third move, you make the life hard for someone who is keen on playing his beloved Queens Gambit Accepted, to name just one example. Without doubt, 1.Nf3 is the most flexible move, depriving the opponent of many options. And flexibility is a hallmark of experts.
Of course, the diagrams don’t show us the results of a qualitative analysis but an overview of empiric data. Can we build our assumption on that foundation? I would say: yes. If you add up the numbers, you find out that we are talking about no less than 9,000,000 games. In my opinion, this huge set of data indeed mirrors reality.
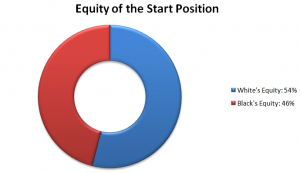
In the start position, White can claim a theoretical ownership of 54% of the full point which is about to be distributed, however, in a more digital way. If two players of equal strength are opposing each other, White can claim a realistic expected value accordingly.
Instead of equity, I also like to use the term “energy”. It is more related to what actually happens on the board. The advantage of the first move loads White’s pieces with 8% more energy, in relation to the 100% of the overall energy of the chess game universe. They can occupy attractive locations more easily and also influence, dominate or threaten their adverse peer group with a higher likelihood.
So, now we can speak it out loud:
The advantage of starting the game amounts to 8% of the overall assets or energy.
By the way, the good thing about empiric data compared to a qualitative analysis is that any kind of psychological factors are already “priced in”. After all, we are dealing with the very results of the battle. I can think of two psychological effects which might very well cancel each other out:
The majority of players play better chess when they feel comfortable and this mostly happens when they have the initiative or advantage. Since White is more likely to have the advantage, he profits more often from this tailwind effect.
However, there is an adverse effect we shouldn’t forget about. My thesis is that if we would examine all positions which are equal between move 15 and 20, Black will score more than 50%. Actually, there might be two different effects responsible for this. The first one is simple. Since White starts with a small advantage, the fact that he couldn’t conserve or even expand it gives a certain bias to the probability that Black is the stronger player. The second effect is less banal.
Many white players feel obliged to prove something and to exploit the advantage of the first move. They feel a bit ashamed to let Black escape with equality. For this reasons, they might tend to still see an advantage, while in reality it already has evaporated. But even if they judge the situation correctly, they might want to make up for their “failure” and turn the tide again by force.
This self-obligation effect is maybe the main reason for the success of some counter openings. I have a lot of experience with the Hedgehog, and I can tell that many of the points that end up on the spines of the beast stem from White players killing themselves. For that reason, it is much less effective to play the Hedgehog with White. The black player, despite having a space advantage would simply sit still behind his wall of pawns and wait for White to do something. After all, a draw with Black can be considered as a small victory. The self-obligation effect is one the reason for the phenomenon that some players actually have better results with the black pieces. They are specialized in the counter style and wait patiently in the trenches with their fingers on the trigger of their machine guns.
I hope you liked my thoughts on the most difficult and most fascinating of all chess positions. The most important thing to remember:
A 54% White score should be the benchmark for the openings we will include in our repertoire.
With White try to aim at 55% or above. And with Black, try to go for 53% or below.
In our world of big data it is not the worst approach to look at chess also as a game of numbers.
